Circles and Spheres

• Arc length surprise. The sum of the areas of the regions between a circular arc and the x-axis, and between the arc and the y-axis, does not depend on the position of the arc! From Mudd Math Fun Facts.

• The Atomium, structure formed for Expo 1958 in the form of nine spheres, representing an iron crystal. The world's largest cube?

• Borromean rings don't exist. Geoff Mess relates a proof that the Borromean ring configuration (in which three loops are tangled together but no pair is linked) can not be formed out of circles. Dan Asimov discusses some related higher dimensional questions. Matthew Cook conjectures the converse.
  

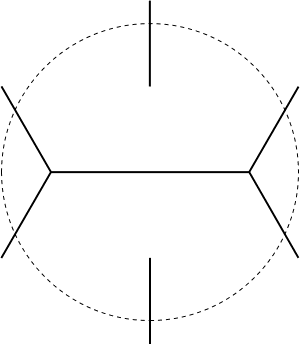
• Building a better beam detector. This is a set that intersects all lines through the unit disk. The construction below achieves total length approximately 5.1547, but better bounds were previously known.
  

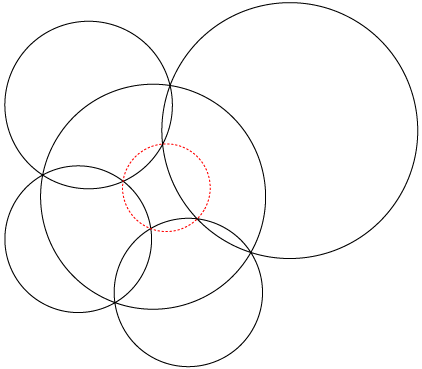
• Circle fractal based on repeated placement of two equal tangent circles within each circle of the figure. One could also get something like this by inversion, starting with three mutually tangent circles, but then the circles at each level of the recursion wouldn't all stay the same size as each other.

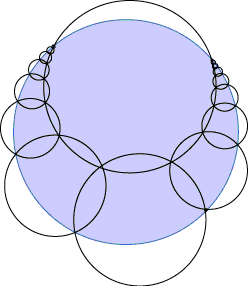
• Circular coverage constants. How big must N equal disks be in order to completely cover the unit disk? What about disks with sizes in geometric progression? From MathSoft's favorite constants pages.
  

• Circular quadrilaterals. Bill Taylor notes that if one connects the opposite midpoints of a partition of the circle into four chords, the two line segments you get are at right angles. Geoff Bailey supplies an elegant proof.

• Circumcenters of triangles. Joe O'Rourke, Dave Watson, and William Flis compare formulas for computing the coordinates of a circle's center from three boundary points, and higher dimensional generalizations.

• Circumference/perimeter of an ellipse: formula(s). Interesting and detailed survey of formulas giving accurate approximations to this value, which can not be expressed exactly as a closed form formula.

• Conceptual proof that inversion sends circles to circles, G. Kuperberg.

• Delaunay and regular triangulations. Lecture by Herbert Edelsbrunner, transcribed by Pedro Ramos and Saugata Basu. The regular triangulation has been popularized by Herbert as the appropriate generalization of the Delaunay triangulation to collections of disks.

• Escher sphere construction kit.

• Filling space with unit circles. Daniel Asimov asks what fraction of 3-dimensional space can be filled by a collection of disjoint unit circles. (It may not be obvious that this fraction is nonzero, but a standard construction allows one to construct a solid torus out of circles, and one can then pack tori to fill space, leaving some uncovered gaps between the tori.) The geometry center has information in several places on this problem, the best being an article describing a way of filling space by unit circles (discontinuously).

• Five circle theorem. Karl Rubin and Noam Elkies asked for a proof that a certain construction leads to five cocircular points. This result was subsequently discovered by Allan Adler and Gerald Edgar to be essentially the same as a theorem proven in 1939 by F. Bath.

• Fractal patterns formed by repeated inversion of circles: Indra's Pearls Inversion graphics gallery, Xah Lee. Inversive circles, W. Gilbert, Waterloo.
  

• Frequently asked questions about spheres. From Dave Rusin's known math pages.

• Hopf fibration. R. Kreminski, the U. Sheffield maths dept., and MathWorld explain and animate the partition of a 3-sphere into circles.

• The HyperSphere, from an Artistic point of View, Rebecca Frankel.

• Hyperspheres. Eric Weisstein calculates volumes and surface areas of hyperspheres, which curiously reach a maximum for dimensions around 5.257 and 7.257 respectively.

• Inversive geometry. Geometric transformations of circles, animated with CabriJava.

• Jiang Zhe-Ming's geometry challenge. A pretty problem involving cocircularity of five points defined by circles around a pentagram.

• The Kneser-Poulsen Conjecture. Bezdek and Connelly solve an old problem about pushing disks together.

• Mathematical imagery by Jos Leys. Knots, Escher tilings, spirals, fractals, circle inversions, hyperbolic tilings, Penrose tilings, and more.

• Minimax elastic bending energy of sphere eversions. Rob Kusner, U. Mass. Amherst.

• Miquel's pentagram theorem on circles associated with a pentagon. With annoying music.

• Miquel's six circles in 3d. Reinterpreting a statement about intersecting circles to be about inscribed cuboids.
  

• Monge's theorem and Desargues' theorem, identified. Thomas Banchoff relates these two results, on colinearity of intersections of external tangents to disjoint circles, and of intersections of sides of perspective triangles, respectively. He also describes generalizations to higher dimensional spheres.

• The Optiverse. An amazing 6-minute video on how to turn spheres inside out.

• Platonic spheres. Java animation, with a discussion of platonic solid classification, Euler's formula, and sphere symmetries.

• Poncelet's porism, the theorem that if a polygon is simultaneously inscribed in one circle and circumscribed in another, then there exists an infinite family of such polygons, one touching each point of each circle. From the secret blogging seminar.

• Pushing disks together. If unit disks move so their pairwise distances all decrease, does the area of their union also decrease?

• Random spherical arc crossings. Bill Taylor and Tal Kubo prove that if one takes two random geodesics on the sphere, the probability that they cross is 1/8. This seems closely related a famous problem on the probability of choosing a convex quadrilateral from a planar distribution. The minimum (over all possible distributions) of this probability also turns out to solve a seemingly unrelated combinatorial geometry problem, on the minimum number of crossings possible in a drawing of the complete graph with straight-line edges: see also "The rectilinear crossing number of a complete graph and Sylvester's four point problem of geometric probability", E. Scheinerman and H. Wilf, Amer. Math. Monthly 101 (1994) 939-943, rectilinear crossing constant, S. Finch, MathSoft, and Calluna's pit, Douglas Reay.

• The reflection of light rays in a cup of coffee or the curves obtained with b^n mod p, S. Plouffe, Simon Fraser U. (Warning: large animated gif. You may prefer the more wordy explanation at Plouffe's other page on the same subject.)

• Vittoria Rezzonico's Java applets. Hypercube and polyhedron visualization, and circle inversion patterns. Requires both Java and JavaScript.

• Sausage Conjecture. L. Fejes Tóth conjectured that, to minimize the volume of the convex hull of hyperspheres in five or more dimensions, one should line them up in a row. This has recently been solved for very high dimensions (d > 42) by Betke and Henk (see also Betke et al., J. Reine Angew. Math. 453 (1994) 165-191 and the MathWorld Sausage Conjecture Page).

• Seven circle theorem, an applet illustrating the fact that if six circles are tangent to and completely surrounding a seventh circle, then connecting opposite points of tangency in pairs forms three lines that meet in a single point, by Michael Borcherds. Other applets by Borcherds include Poncelet's porism, a similar porism with an ellipse and a parabola and with two ellipses, and more generally with two conics of variable type.

• Sierpinski carpet on the sphere. From Curtis McMullen's math gallery.

• Sliced ball. Ray-traced image created to help describe recent algorithms for removing slivers from tetrahedral meshes.
  

• Soccer ball pictures, spherical patterns generated by reflections that form rational angles to each other.

• Sphere packing and kissing numbers. How should one arrange circles or spheres so that they fill space as densely as possible? What is the maximum number of spheres that can simultanously touch another sphere?

• Tangencies. Animated compass and straightedge constructions of various patterns of tangent circles.
  

• Tangencies of circles and spheres. E. F. Dearing provides formulae for the radii of Apollonian circles, and analogous three-dimensional problems.

• Tetrahedrons and spheres. Given an arbitrary tetrahedron, is there a sphere tangent to each of its edges? Jerzy Bednarczuk, Warsaw U.

• Morwen Thistlethwait, sphere packing, computational topology, symmetric knots, and giant ray-traced floating letters.

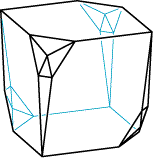
• An uninscribable 4-regular polyhedron. This shape can not be drawn with all its vertices on a single sphere.
  

• Visualizing the hypersphere via 3d slices, and other four-dimensional thoughts by Jeff Fuquay.

• WWW spirograph. Fill in a form to specify radii, and generate pictures by rolling one circle around another. For more pictures of cycloids, nephroids, trochoids, and related spirograph shapes, see David Joyce's Little Gallery of Roulettes. Anu Garg has implemented spirographs in Java.

• yukiToy. Shockwave plugin software for pushing around a few reddish spheres in your browser window. But what exactly is the point? (They're spheres, they don't have one, I guess.)

From the Geometry Junkyard, computational and recreational geometry pointers.
Send email if you know of an appropriate page not listed here.
David Eppstein, Theory Group, ICS, UC Irvine.
Semi-automatically filtered from a common source file.